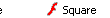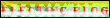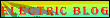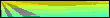For many conductors of electricity, the electric current which will flow through them is directly proportional to the voltage applied to them. When a microscopic view of Ohm's law is taken, it is found to depend upon the fact that the drift velocity of charges through the material is proportional to the electric field in the conductor. The ratio of voltage to current is called the resistance, and if the ratio is constant over a wide range of voltages, the material is said to be an "ohmic" material. If the material can be characterized by such a resistance, then the current can be predicted from the relationship:
 The AC analog to Ohm's law is
The AC analog to Ohm's law is
 where Z is the impedance of the circuit and V and I are the rms or effective values of the voltage and current. Associated with the impedance Z is a phase angle, so that even though Z is the also the ratio of the voltage and current peaks, the peaks of voltage and current do not occur at the same time. The phase angle is necessary to characterize the circuit and allow the calculation of the average power used by the circuit.
where Z is the impedance of the circuit and V and I are the rms or effective values of the voltage and current. Associated with the impedance Z is a phase angle, so that even though Z is the also the ratio of the voltage and current peaks, the peaks of voltage and current do not occur at the same time. The phase angle is necessary to characterize the circuit and allow the calculation of the average power used by the circuit.
 If an rms voltage of Vrms =380
If an rms voltage of Vrms =380
is applied to an impedance Z = 10 ohms,
then the rms current will be Irms = 38 A.
If the phase is φ = 3 degrees,
then the power factor is cosφ = 0.9986
and the average power is
Pavg = VrmsIrmscosφ = 14420.21 watts.
 The illustration is for a case where the inductive reactance is dominant over the capacitive reactance as shown in the phasor diagram.
The illustration is for a case where the inductive reactance is dominant over the capacitive reactance as shown in the phasor diagram.
Default values will be entered for V and Z above is they are left unspecified, but those values can be changed. If the current is changed, then Z will be recalculated. If a phase angle outside the allowed range -90 to +90 is entered, it will be replaced by a default value.
 The AC analog to Ohm's law is
The AC analog to Ohm's law is where Z is the impedance of the circuit and V and I are the rms or effective values of the voltage and current. Associated with the impedance Z is a phase angle, so that even though Z is the also the ratio of the voltage and current peaks, the peaks of voltage and current do not occur at the same time. The phase angle is necessary to characterize the circuit and allow the calculation of the average power used by the circuit.
where Z is the impedance of the circuit and V and I are the rms or effective values of the voltage and current. Associated with the impedance Z is a phase angle, so that even though Z is the also the ratio of the voltage and current peaks, the peaks of voltage and current do not occur at the same time. The phase angle is necessary to characterize the circuit and allow the calculation of the average power used by the circuit. If an rms voltage of Vrms =380
If an rms voltage of Vrms =380is applied to an impedance Z = 10 ohms,
then the rms current will be Irms = 38 A.
If the phase is φ = 3 degrees,
then the power factor is cosφ = 0.9986
and the average power is
Pavg = VrmsIrmscosφ = 14420.21 watts.
 The illustration is for a case where the inductive reactance is dominant over the capacitive reactance as shown in the phasor diagram.
The illustration is for a case where the inductive reactance is dominant over the capacitive reactance as shown in the phasor diagram.Default values will be entered for V and Z above is they are left unspecified, but those values can be changed. If the current is changed, then Z will be recalculated. If a phase angle outside the allowed range -90 to +90 is entered, it will be replaced by a default value.
Labels: Basic electric
0 Comments:
Subscribe to:
Post Comments (Atom)